Post-spinel transition in Mg2SiO4
Purpose of this study
- Studying detailed phase relations of post-spinel transition in Mg2SiO4
-
- Checking the results of Ito & Takahashi (1989) and Irifune et al. (1998) by in situ X-ray diffraction in a multi-anvil apparatus
- At what pressure is the phase boundary located at the realistic mantle temperature?
- How is the Clapeyron slope of the post-spinel transition?
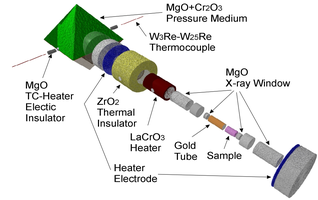
Experimental setup
- The starting material: mixture of Mg2SiO4 + Au.
- X-rays are incidented in parallel to the axis of the cylindrical heater
- P is calculated from T and unit cell volume of Au using EOS of Au by Anderson et al. [1989].
Main difficulty and its solution
- Inertness of ringwoodite
-
- Difficult to initiate dissociation of ringwoodite into perovskite + periclase
-
- Especially when annealed at HT for a long time.
- Crystalline perfection increases by annealing
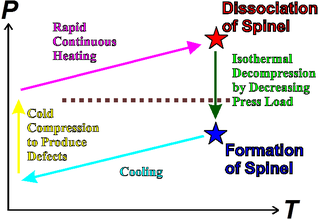
- Solution: rapid heating
-
- 100-1000 K/min
- thermal shock
-
- May create dislocations in spinel grains
- Once initiated, the reaction completes within a few minutes.
-
- very fast
Examples of change in diffraction pattern
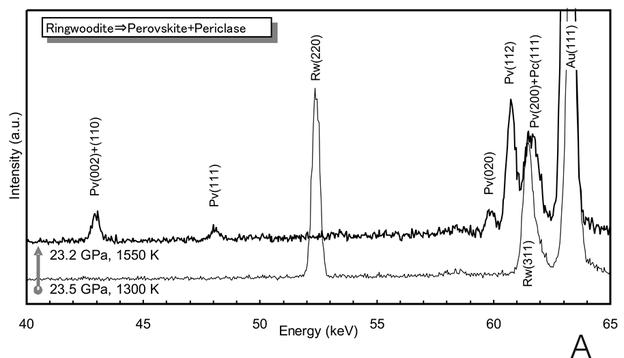
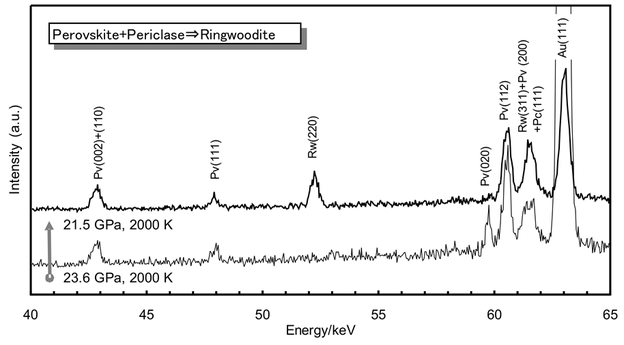
Experimental results
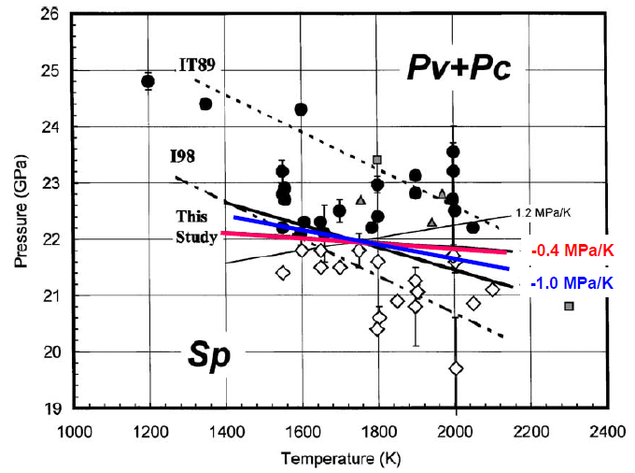
Discussion
- P at 660-km discontinuity is 23 GPa, whereas P of the post-spinel transition observed in this study is 22 GPa.
-
- 1 GPa higher than that of Irifune et al. (1998)
- Still 1 GPa lower than 660-km discontinuity
-
- Because of uncertainty of EOS of Gold?
- 5% error is possible
- Thermal pressure and/or pressure derivative of bulk modulus
- Small temperature dependence of post-spinel transition
-
- If steep negative slope (>|-2| MPa/K), the mantle convection can be prevented on the boundary
- Post spinel transition cannot be a barrier of the mantle convection.
- Completely against the previous understanding!
Successive studies
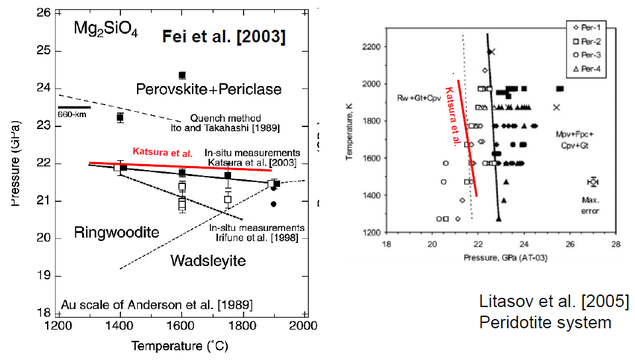
People doubted the results of this study, and the phase relation were repeatedly studied [cf. Fei et al., 2004; Litasov et al., 2005].
But the results are the same. Very small Clapeyron slope!
- If dP/dT = -0.4 MPa/K → Ca. 1 km/100K
-
- ±20 km topography → ±2000 K
-
- Too large variation
- The temperature at 660 km depth : ~2000K
- If dP/dT = -1.0 MPa/K → Ca. 2.5 km/100K
-
- ±20 km topography → ±800 K
- Still very large
- Temperature dependence of seismic wave velocity → 0.6 % / 100 K
- Purturbation of seismic wave velocity : ±2 % → ± 300 K variation in temperature
- Temperature variation inferred from the topography of 660-km discontinuity is too large (±800 K)
- The temperature around 660 km depth is supposed to be 2000 K (1700℃)
- If there is temperature variation of ±800 K, high temperature part of the mantle should melt.
- However, mantle is solid, because shear wave propagates in the mantle
- We cannot accept temperature variation of ±800 K. We need some other factors to increase topology of the 660 km discontinuity
Conclusion
- By combination of multi-anvil high-pressure experiment and synchrotron-based X-ray diffraction, it was found that the slope of breakdown of ringwoodite to perovskite + periclase is very shallow.
- It is difficult to explain topography of the 660-km discontinuity and slab stagnation.
- The negative slope is not a reason for the slab stagnation. Other factors should be important. However, there are no definite answer for them at present.
