Why squared difference in length?
When the Eulerian finite strain is derived, the argument started from change in squared length of an object. I do not understand why change in squared length is essential. However, let me anyway discuss what equation of states are obtained if change in linear and cubed length are considered.
Note that the geometrical setting and notations in this page are identical to those in the page of "Finite Strain". FS refers to the pages explaining the finite strain, and BM2 refers to the page explaining the 2nd-order Birch-Murnaghan EOS.
Linear difference
In this scheme, we just consider difference of length, unlike the square difference in the usual finite strain theory.
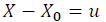
(1)
We use the linear relation between u and X (Eq. 5 in FS), and define the (finite) strain by:
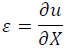
(2)
Eq (1) is expressed by:
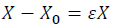
(3)
Then, we express the (finite) strain by the volume ratio in the same way as the squared case.
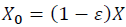
(4)
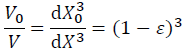
(5)
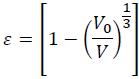
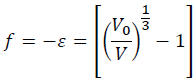
The V derivative of f is:
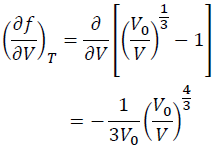
The f and its V derivative, and parameter a are substituted into Eq. (4) in BM2, and we have:

Finally, we obtain the 2nd-order EOS based on the linear length difference:
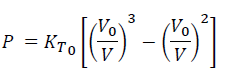
Cubed difference
In this scheme, we just consider cubed difference of length, unlike the square difference in the usual finite strain theory.
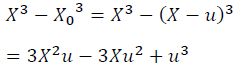
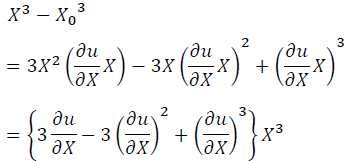
We use the linear relation between u and X (Eq. 5 in FS), and define the finite strain by:
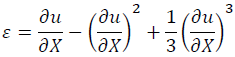
(13)
Then, we express the finite strain by the V ratio in the same way as the squared case.
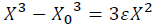
(14)
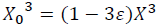
(15)
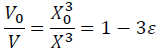
(16)
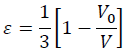
(17)

(18)
The V derivative of f is:
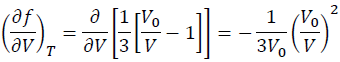
(19)
The f and its V derivative, and parameter a are substituted into Eq. (4) in BM2, and we have:
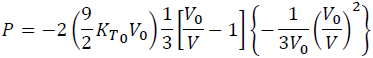
(20)
Finally, we obtain the 2nd-order EOS based on the cubed length difference:
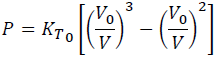
(21)
Summary
Let us summarize the finite strains, its volume derivatives, and pressures by 2nd-order EOS's in the case of linear, square and cubed differences are considered.
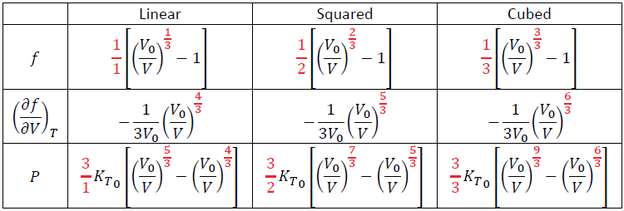
Differences among these three cases are as follows. Let be n = 1, 2, 3 for the linear, squared and cubed cases, respectively.
(1) The finite strains are proportional to the n/3 power of the V ratio.
(2) The coefficients to the finite strains are 1/n.
(3) The V derivatives of the finite strain are proportional to the (1+n/3) power of the V ratio.
(4) P by the EOS's are proportional to difference in the (2n+3)/3 and (n+3)/3 powers of the V ratio.
(5) The coefficients at the top of the EOS's are 3/n.
These features imply that energy is more rapidly stored in a matter with compression in the higher power of length. This fact is clearly shown by pressures obtained by these three case as shown in Fig. 1 (below).
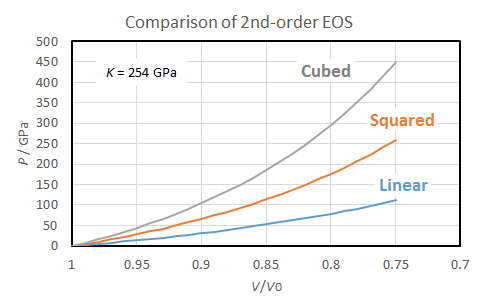
Fig. 1
As is known, the EOS based on the squared difference in length gives proper pressure. Therefore EOS's based on the linear and cubed differences in length give too low and too high pressures, respectively. Again, I do not know why the EOS baed on the squared difference in length gives proper values. However, experimental results support it.
If you know the physical reason why the squared length difference is essential, please let me know it.
